The area of the region where their paths overlap is ${288\over 83}\text{ ft}^2 \approx 3.47 \text{ ft}^2$.
In our solution, we will use the following notational convention
$$XY = \text{ the length of the side joining } X \text{ and } Y.$$ We will take advantage of various proportions that result from similar triangles found within the figure below.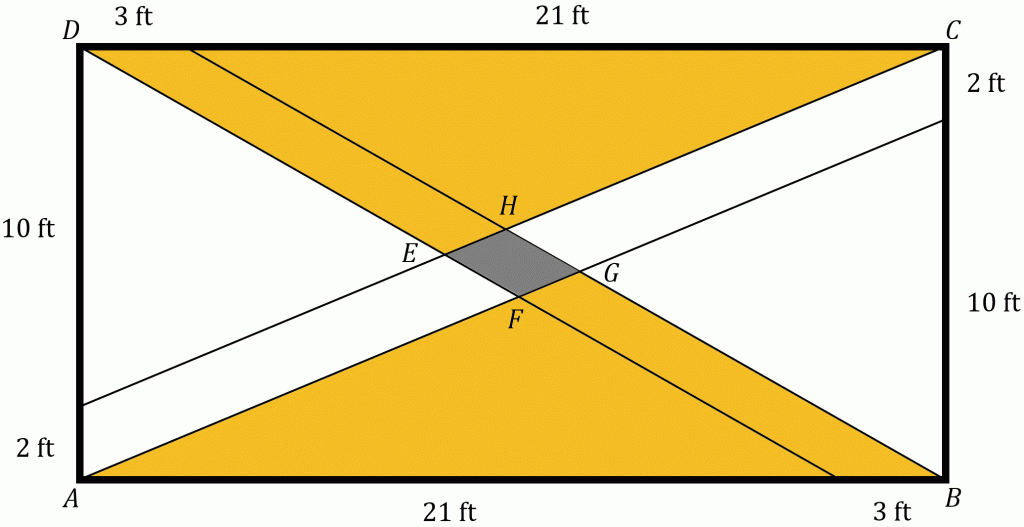
The two orange colored triangles ($\triangle ABG \text{ and } \triangle CDE$) can be combined together to form a parallelogram that shares an angle in common with parallelogram $EFGH$. In particular, we have $\angle AGB = \angle EHG$. Similarly, the two white colored triangles ($\triangle ADF$ and $\triangle BCH$) can be combined together to form a parallelogram that shares an angle in common with parallelogram $EFGH$. In particular, we have $\angle AFD = \angle FGH$. See the figure below.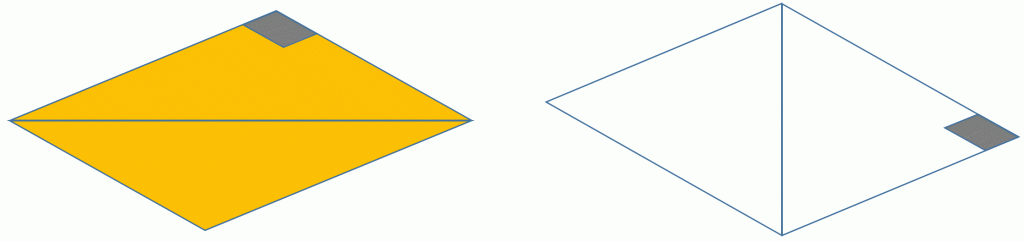
Using properties of similar triangles, we obtain the following relationships between the sides of parallelogram $EFGH$ and the corresponding sides of the parallelograms formed by the orange and white triangles. $\hskip.5in {AG\over FG}={24\over 3} \hskip.2in \text{and therefore, } AG=8(FG)=8(EH) \\ \hskip.5in {BG\over GH}={10\over 2} \hskip.2in \text{therefore, } BG=5(GH) \\ \hskip.5in {DF\over EF}={12\over 2} \hskip.2in \text{and therefore, } DF=6(EF)=6(HG) \\ \hskip.5in {AF\over FG}={21\over 3} \hskip.2in \text{therefore, } AF=7(FG) $
If we let $P$ denote the area of parallelogram $EFGH$, it follows that: $$\text{Area of the parallelogram formed by the orange triangles } = 8(5)P = 40P \\ \text{Area of the parallelogram formed by the white triangles } = 6(7)P = 42P$$The area of the entire rectangular driveway is (24 ft)(12 ft) = 288 $\text{ft}^2$ which can be broken up as the sum of the areas of the orange triangular regions, the white triangular regions, and the parallelogram representing the overlapping paths. Using this fact and our results above, we have $$40P+42P+P=288 \hskip.2in \text{ and thus, } \hskip.2in P={288\over 83}\approx 3.47$$
